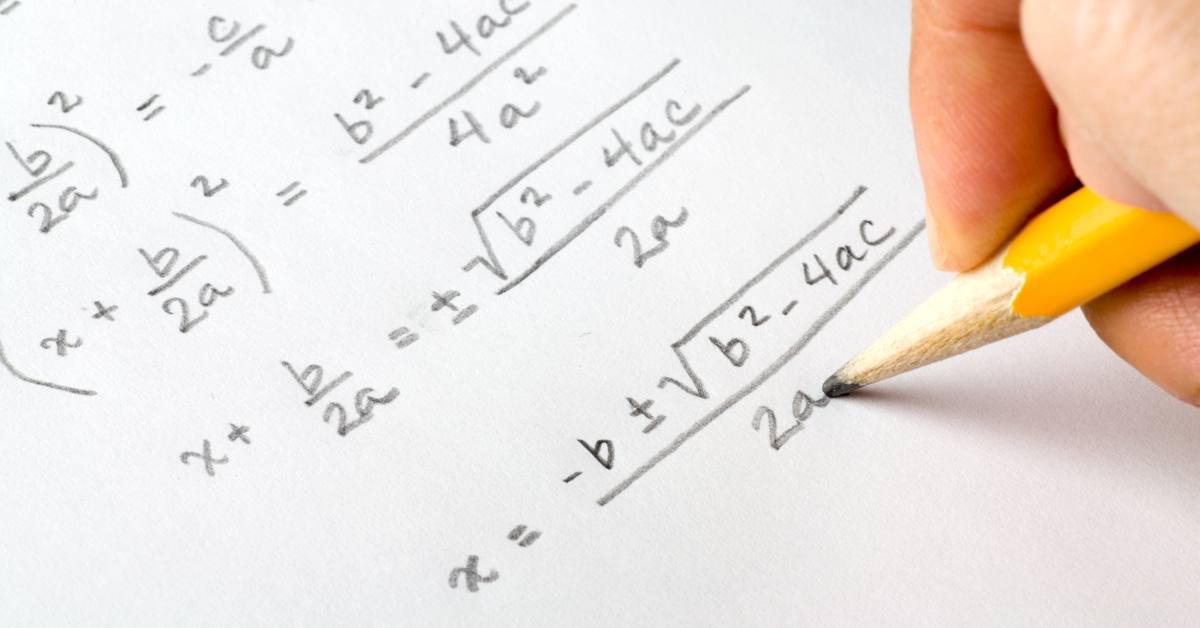Introduction to Algebra: Essential Terminology and Techniques
Algebra is not just a subject within mathematics; it's a foundational tool that enhances logical thinking and problem-solving abilities across various disciplines. Whether it's for academic purposes or everyday problem-solving, mastering introductory algebra techniques is essential. Algebra helps in understanding the relationships between numbers, and it serves as a gateway to more advanced mathematical concepts. From enhancing critical thinking skills to improving one's ability to reason logically, the impact of algebra extends beyond the classroom.
Essential Terminology in Algebra
Variables, Constants, and Coefficients
These three are the alphabets of the algebraic language. A variable represents an unknown value that can change depending on the context of the problem, symbolized by letters such as x, y, or z. Constants are fixed values that do not change, like the numbers 3, -5, or 100. Coefficients are the numbers that multiply the variables, indicating their strength or frequency in an equation. Together, these elements help express mathematical ideas in a concise and manageable way, paving the path for effective basic algebra principles application.
Using Terms and Expressions
In algebra, terms are the building blocks of expressions and equations, consisting of numbers, variables, and their combinations through multiplication or division. An expression, on the other hand, is a group of terms combined using addition or subtraction operations without an equals sign. Learning how to properly define and manipulate these terms and expressions is crucial for setting up and solving algebraic problems. This skill is fundamental in ensuring that students can transition smoothly from simple calculations to more complex algebra problem-solving scenarios.
Key Algebraic Structures
Functions, inequalities, and absolute values are foundational concepts in algebra that enable students to understand and solve a wide array of mathematical problems. A function is a relation between a set of inputs and a set of permissible outputs, ensuring that each input is related to exactly one output. This one-to-one correspondence is crucial for mathematical analysis and problem-solving, as it allows for the creation of predictable and consistent results. Functions can be represented in various forms, such as equations, graphs, or tables, and can describe phenomena ranging from simple linear relationships to complex non-linear dynamics found in fields like economics and physics. They are particularly important in calculus, where they help define limits, derivatives, and integrals, which are critical for modeling continuous change.
Inequalities are expressions that compare the relative sizes or values of two objects using symbols such as < (less than), > (greater than), ≤ (less than or equal to), or ≥ (greater than or equal to). They are essential for defining ranges of solutions and conditions within a problem, allowing mathematicians and scientists to express constraints and parameters necessary for decision-making and theoretical research. For example, inequalities are fundamental in optimization problems where one seeks to maximize or minimize a particular function subject to certain constraints.
Absolute values quantify the magnitude of a number on a numerical scale without considering its direction from zero. This concept is critical not only in pure mathematics but also in real-world applications such as engineering and computer science. In geometry, the absolute value can represent distances between points, while in finance, it helps measure absolute differences in stock prices, ignoring whether they have moved up or down. Moreover, absolute values facilitate the understanding of complex numbers, providing a measure of their magnitude regardless of their direction in the complex plane.
Importance of the Order of Operations
It dictates the sequence in which operations should be performed within an expression to achieve the correct result. Known by the acronym PEMDAS (Parentheses, Exponents, Multiplication and Division, Addition and Subtraction), this rule ensures that all mathematicians speak the same language when calculating. A solid grasp of this order is essential for anyone looking to master algebra math tutor skills, as it prevents common mistakes and promotes accuracy in solving problems.
Basic Algebra Techniques
Solving Linear Equations
These equations involve variables raised to the first power and do not contain variables multiplying each other. The primary goal is to isolate the variable on one side of the equation to determine its value. This involves inverse operations such as adding or subtracting the same number from both sides of the equation and multiplying or dividing by a coefficient. Mastery of these techniques allows students to resolve real-world problems, such as calculating distances or predicting outcomes, reinforcing the practical utility of solving algebra equations.
Techniques for Solving Systems of Equations
Systems of equations consist of multiple equations that share the same set of variables. Solving these systems requires finding the values of the variables that satisfy all equations simultaneously. Here are the key techniques used:
Substitution Method: For example, if one equation is solved for x in terms of y, this expression for x is substituted into the other equations. This approach transforms the system into a simpler one, usually reducing the number of equations or the number of variables in each equation. The substitution method is particularly effective for small systems or systems where one equation can be easily isolated for one variable.
Elimination Method: This technique often involves multiplying one or both equations by a number to align coefficients for one of the variables, allowing that variable to be eliminated when the equations are combined. By breaking down the system into smaller variables, this approach facilitates the solution of the remaining system through additional substitution or elimination.
Graphical Method: This method requires plotting each equation as a graph, typically as lines in two-dimensional space or planes in three-dimensional space. The solution to the system of equations corresponds to the points where the graphs intersect. This method provides a visual representation of the solutions and is useful for understanding the relationship between the equations. However, it is less precise for systems that do not have integer solutions or for systems in higher dimensions.
Matrix Method: Also known as the method of row reduction or Gaussian elimination, this technique uses matrix operations to simplify the system into a form that is easier to solve. By representing the system of equations as an augmented matrix, operations are performed to reduce this matrix to row echelon form or reduced row echelon form, from which the solutions can be directly read or easily deduced.
Each of these methods offers different advantages and is suitable for different types of systems, ensuring that mathematicians and scientists have a variety of tools at their disposal to tackle various systems of equations.
Approaching Polynomial Equations
Polynomial equations involve terms with variables raised to positive integer powers. To solve these equations, one must understand how to rearrange and simplify terms effectively. Techniques such as factoring the polynomial, using the rational root theorem, or applying synthetic division are pivotal. Strategies such as these help break down higher-degree polynomials into simpler linear or quadratic factors, facilitating easier solutions and bolstering skill sets.
Using the Distributive Property and Factoring
The distributive property allows you to multiply a sum or difference by multiplying each addend separately and then adding or subtracting the results. It is vital in simplifying algebraic expressions and solving equations involving parentheses. Conversely, factoring involves breaking down a complex expression into simpler multipliers that, when multiplied together, reproduce the original expression.
Role of Algebra Tutors
Benefits of Personalized Instruction
Personalized instruction provided by algebra tutors can significantly enhance a student's understanding and mastery of algebra. This customized approach is particularly beneficial in a subject that many students find challenging. Here are some of the key benefits:
Targeted Learning: Tutors can pinpoint exactly where a student may be struggling, such as with quadratic equations or logarithmic functions. By focusing specifically on these areas, sessions can be highly effective in overcoming obstacles. The tutor assesses the student’s current knowledge and continuously adapts the lessons to focus on the most relevant areas, ensuring that each session builds upon the last and is tailored to the student's specific challenges.
Flexible Pacing: Each student learns at a different pace, and personalized instruction respects this variability. For students who grasp concepts quickly, the tutor can accelerate the pace of learning, introducing more advanced topics earlier. If a student needs more time to understand certain concepts, the tutor can slow down and provide additional practice.
Immediate Feedback: In personalized tutoring sessions, students benefit from immediate feedback on their work. This allows them to understand exactly what errors they made, learn why they made them, and how to correct them on the spot. Such feedback is invaluable as it helps prevent the reinforcement of incorrect practices and clears up misunderstandings immediately, improving learning efficiency and effectiveness.
Increased Engagement: Tutoring sessions that are tailored to the student’s interests and learning style can make a significant difference in their engagement levels. Engaged students are more likely to participate actively and retain information better, which is crucial in mastering a complex subject.
Boosted Confidence: When students receive focused, personal attention in their learning, they often experience a boost in confidence. This is especially true in subjects like algebra, where concepts build on each other progressively. As students see their progress and understand that they can solve problems on their own, their confidence grows. This newfound confidence can encourage them to explore more challenging aspects of algebra and other mathematical areas with enthusiasm and less anxiety.
With tailored support and guidance from a tutor, students can navigate the complexities of algebra with greater ease and confidence, leading to better overall academic performance and a more positive attitude toward learning.
Finding an Algebra 1 Tutor
Seeking an Algebra 1 tutor is ideal for students at the beginning of their algebra journey. These tutors specialize in foundational skills that are crucial for future success in mathematics. They focus on basic concepts such as solving linear equations, understanding variables, and mastering the order of operations. A proficient tutor will ensure that a student builds a strong base, preparing them adequately for more advanced studies in algebra.
Algebra 2 Tutors for Advanced Topics
As students progress, algebra 2 tutors become invaluable in navigating more complex topics such as functions, polynomials, and exponential equations. These tutors help students delve deeper into the subject, enhancing their understanding and preparing them for higher-level courses. The advanced expertise of these tutors is crucial for students who need to solidify their algebraic skills and ensure readiness for future challenges in calculus and other higher mathematics.
Linear Algebra Tutors for Specialized Coursework
Linear algebra tutors offer specialized instruction in areas involving vectors, matrices, and systems of equations. This branch of algebra is essential for students pursuing studies in engineering, physics, and computer science. A tutor with expertise in linear algebra can provide critical insights and problem-solving techniques that are not typically covered in standard algebra courses, thus equipping students with the necessary tools to succeed in their respective fields.
Practical Tips and Resources
Importance of Consistent Practice
Regular engagement with algebraic concepts solidifies understanding and helps students develop a quicker, more accurate approach to problem-solving. It's important to vary the complexity and type of problems to cover all aspects of fundamental algebra skills.
Finding Reliable Tutors and Resources
Resources such as online platforms, tutoring centers, and academic websites offer access to qualified tutors. Additional material can be found in resources like textbooks, educational websites, and apps, which can support and reinforce lessons that are taught in class or by tutors.
The journey through algebra is not just about acquiring mathematical skills but also about developing critical thinking and problem-solving abilities. From basic operations to complex equations, algebra teaches us to analyze, interpret, and solve problems effectively. Personalized abstract algebra tutoring plays a pivotal role in this educational journey, offering tailored instruction that meets individual learning needs and paces. It bridges gaps in understanding, fosters deeper comprehension, and builds confidence, preparing students not only for advanced mathematics but for analytical challenges across diverse fields. Whether navigating the fundamentals of Algebra 1 or tackling the complexities of linear algebra, effective learning strategies, and the right support can make a significant difference in a student's academic and professional future. Embracing these educational tools and resources is essential for anyone looking to master algebra and leverage its benefits for lifelong success.